Higher-order topology in bismuth

Abstract

The mathematical field of topology has become a framework in which to describe the low-energy electronic structure of crystalline solids. Typical of a bulk insulating three-dimensional topological crystal are conducting two-dimensional surface states. This constitutes the topological bulk–boundary correspondence. Here, we establish that the electronic structure of bismuth, an element consistently described as bulk topologically trivial, is in fact topological and follows a generalized bulk–boundary correspondence of higher-order: not the surfaces of the crystal, but its hinges host topologically protected conducting modes. These hinge modes are protected against localization by time-reversal symmetry locally, and globally by the three-fold rotational symmetry and inversion symmetry of the bismuth crystal. We support our claim theoretically and experimentally. Our theoretical analysis is based on symmetry arguments, topological indices, first-principles calculations, and the recently introduced framework of topological quantum chemistry. We provide supporting evidence from two complementary experimental techniques. With scanning-tunnelling spectroscopy, we probe the signatures of the rotational symmetry of the one-dimensional states located at the step edges of the crystal surface. With Josephson interferometry, we demonstrate their universal topological contribution to the electronic transport. Our work establishes bismuth as a higher-order topological insulator.

Main

Electronic insulators are characterized by an energy gap between valence and conduction bands. Two insulators are classified as topologically equivalent, if one can be deformed to the other without closing this gap, while certain symmetries are respected. If time-reversal symmetry (TRS) is respected in this deformation process, three-dimensional insulators have been shown to fall into two disconnected topological classes: trivial and nontrivial^{1,2,3,4,5,6,7}. The latter are called topological insulators. What makes this mathematical classification highly relevant experimentally is the so-called bulk–boundary correspondence of topological insulators: the two-dimensional surface of a three-dimensional topological insulator hosts conducting states, with the dispersion of a non-degenerate Dirac cone, that cannot be gapped or localized without breaking TRS (or inducing interacting instabilities such as superconductivity or topological order). When, in addition, the spatial symmetries of the crystal are preserved during this deformation process, such as mirrors or rotations, one may speak of topological crystalline insulators^{8,9,10,11,12,13}. The additional symmetries have been argued to stabilize multiple Dirac cones on surfaces that are invariant under both TRS and the protecting spatial symmetry.

More recently, topological crystalline insulators were generalized to also include higher-order topological insulators (HOTIs)^14,15, in which gapless topological states protected by spatial symmetries appear at corners or hinges, while the edges and surfaces are gapped in two-dimensional and three-dimensional systems, respectively. HOTIs thus generalize the topological bulk–boundary correspondence. Whereas the topological protection of point-like corner modes requires some spectral symmetry, one-dimensional gapless hinge modes mediate a spectral flow^15,16,17,18 between valence and conduction band of the bulk insulator, akin to quantum Hall^19,20,21,22 or quantum spin Hall edge modes^{23,24,25,26,27,28,29,30}. Therefore, they can be expected to appear more generically in true crystalline materials. Several works studied the classification of HOTIs^{16,18,31,32,33,34,35,36}, for example in the presence of two-fold spatial symmetries¹⁶ or $\hat C_n$ rotational symmetries³⁷.

Various topological aspects of the electronic structure of bismuth have been studied experimentally in the past. This revealed intriguing features such as one-dimensional topological modes localized along step edges on the surface of bismuth³⁸, conducting hinge channels on bismuth nanowires^39,40, quasi-one-dimensional metallic states on the bismuth (114) surface⁴¹, and a quantum spin Hall effect in two-dimensional bismuth bilayers^42,43 and bismuthene on silicon carbide⁴⁴. Here we show, using band representations and the theory of topological quantum chemistry^{45,46,47,48,49,50}, that bismuth is in fact a HOTI. This finding provides a unified theoretical origin for all these previous experimental observations. The crystal symmetries that protect the topology of bismuth, $\hat C_3$ rotation and inversion, establish a new class of HOTIs not discussed in previous works^{14,15,16,17,18,31,32,33,34,35,36,51}. We support our theoretical analysis with experimental data using two complementary techniques: scanning tunnelling microscopy/spectroscopy (STM/STS) on surface step edges and Josephson interferometry on bismuth nanowires.

Another three-dimensional material that hosts one-dimensional modes on its surface is the topological crystalline insulator tin telluride. Strained tin telluride is proposed to be a HOTI¹⁵. In addition, tin telluride has been experimentally shown to feature one-dimensional flatband modes localized at surface step edges⁵². The latter appear together with the Dirac cone topological surface states and are thus distinct from the hinge modes of a HOTI.

While there are analogous experimental realizations of two-dimensional second-order topological insulators via electrical circuits⁵³, as well as phononic⁵⁴ and photonic⁵⁵ systems, this work provides a realization of a three-dimensional HOTI in the electronic structure of a crystal.

Bulk topology

Fu and Kane⁵ gave a simple topological index for a three-dimensional topological insulator in the presence of inversion symmetry $\hat{\rm {I}}$: One multiplies the inversion eigenvalues (which are ±1) of all Kramers pairs of occupied bands at all time-reversal symmetric momenta in the Brillouin zone. If this product is −1 (+1), the insulator is topological (trivial). In the topological case, one says the material has a band inversion. Note that when we evaluate this index for bismuth, we obtain +1, in accordance with the well known result that the band structure of bismuth is topologically trivial from a first-order perspective⁵⁶. A sample of bismuth thus does not have topologically protected gapless surface states. However, this is not because bismuth does not display a band inversion: we will show that there are two band inversions, whose presence is not captured by the first-order index, which is only sensitive to the parity of band inversions. We first extend this index to HOTIs with TRS, $\hat C_3$ rotation, and inversion symmetry $\hat I$. We consider a $\hat C_3$ rotational symmetry with axis that is given by the line connecting the time-reversal symmetric momenta Γ and T (see Fig. 1a for a representation of the Brillouin zone). For spin-1/2 particles, $\hat C_3$ has eigenvalues −1 and exp(±iπ/3), where a subspace with −1 eigenvalue is closed under TRS, while TRS maps the exp(±iπ/3) subspace to the exp(±iπ/3) one and vice versa. We can thus define a band inversion separately in the occupied band subspaces of an insulator with $\hat C_3$ eigenvalues −1 and exp(±iπ/3). To do so, observe that of the eight time-reversal symmetric momenta, two are invariant under $\hat C_3$ (Γ and T), while two groups of three time-reversal symmetric momenta transform into each other under $\hat C_3$ (call them X_i and L_i, i = 1, 2, 3). Denote by $\nu _{\rm{Y}} = \mathop {\prod}\nolimits_{i \in {\mathrm{occ}}} \xi _{i,{\rm{Y}}}$ the product over all inversion eigenvalues ξ_i,Y = ±1 of the occupied bands’ Kramers pairs at the time-reversal symmetric momenta ${\rm{Y}} \in \{ {{\Gamma}} ,{\rm{T}},{\rm{X}}_i,{\rm{L}}_i\}$. At Γ and T we further define $\nu _{\rm{Y}}^{(\pi )}$ and $\nu _{\rm{Y}}^{( \pm \pi /3)}$, where the product is restricted to the Kramers pairs with $\hat C_3$ eigenvalues −1 and exp(±iπ/3), respectively, such that $\nu _{\rm{Y}} = \nu _{\rm{Y}}^{(\pi )}\nu _{\rm{Y}}^{( \pm \pi /3)}$ for Y = Γ, T. By $\hat C_3$ symmetry $\nu _{{\rm{X}}_1} = \nu _{{\rm{X}}_2} = \nu _{{\rm{X}}_3}$ and $\nu _{{\rm{L}}_1} = \nu _{{\rm{L}}_2} = \nu _{{\rm{L}}_3}$, so that the Fu–Kane index is given by $\nu = \nu _{{\Gamma}} \nu _{\rm{T}}\nu _{{\rm{X}}_1}\nu _{{\rm{L}}_1}$. Consider a Kramers pair of states at X₁ together with its two degenerate $\hat C_3$ partners at X₂ and X₃. Out of a linear combination of these states, one can construct one Kramers pair with $\hat C_3$ eigenvalue −1, and two Kramers pairs with eigenvalues exp(±iπ/3). This is shown explicitly in the Supplementary Information. When taking the Kramers pair at X₁ together with its degenerate partners at X₂ and X₃ to have negative inversion eigenvalue, these $\hat C_3$ symmetric linear combinations also have negative inversion eigenvalue. Thus, a band inversion at X_i as measured by the Fu–Kane formula induces a single band inversion in the −1 subspace, and two (which equals no) band inversions in the exp(±iπ/3) subspace. The same holds for the L_i points. We conclude that the total band inversion in the occupied subspaces with $\hat C_3$ eigenvalues −1 and exp(±iπ/3) are given by

$$\nu ^{(\uppi )} = \nu _{{\Gamma}} ^{(\uppi )}\nu _{\rm{T}}^{(\uppi )}\nu _{{\rm{X}}_1}\nu _{{\rm{L}}_1},\quad \quad \nu ^{( \pm \uppi /3)} = \nu _{{\Gamma}} ^{( \pm \uppi /3)}\nu _{\rm{T}}^{( \pm \uppi /3)}$$

(1)

respectively. We then distinguish three cases: (i) v^(π) = v^(±π/3) = +1 for a trivial insulator, (ii) v = v^(π)v^(±π/3) = −1 for a ${\Bbb Z}_2$ topological insulator, and (iii) v^(π) = v^(±π/3) = −1 for a HOTI.

Fig. 1: Electronic structure of a HOTI with $\hat C_3$ and $\hat I$.

a, Brillouin zone with TRS points that are used to evaluate the topological indices in equation (1). b, Unit cell of the crystal structure of bismuth, which has $\hat C_3$ and inversion symmetry. There are six inequivalent sites in the conventional (hexagonal) unit cell, which is shown in red. Black lines delineate the primitive unit cell (rhombohedral), which has only two inequivalent atoms. c, Schematic of the hinge states of a hexagonally shaped HOTI oriented along the trigonal [111] axis, with $\hat C_3$ and inversion symmetry (such as bismuth). We note that a prism with triangular rather than hexagonal cross-section would not respect inversion symmetry. All edges of the hexagonal cross-section are along bisectrix axes. Red lines represent a single one-dimensional Kramers pair of gapless protected modes. In the Dirac picture of a HOTI surface, red and blue surfaces correspond to opposite signs of the unique TRS surface mass term. d, Localized hinge modes of the minimal tight-binding model of a HOTI with the same topology and symmetries as bismuth, as defined in the Supplementary Information. The model is solved on the hexagon geometry described in c with open boundary conditions in all directions. Plotted is the sum of the absolute squares of the eigenstates that lie in the bulk and surface gap. Although the tight-binding model considered has the same topology as bismuth, it lacks its metallic surface states, which are not protected by $\hat C_3$ and inversion symmetry. e, Band structure of bismuth with inversion eigenvalues (green) and $\hat C_3$ eigenvalues on the Γ–T line (black). E_F denotes the Fermi energy. Since valence bands (red) and conduction bands (blue) are not degenerate anywhere in momentum space, their topological indices (see equation (1)) are well defined despite the appearance of a small electron and hole pocket. Black arrows indicate the two valence bands contributing to the $\hat C_3$-eigenvalue-graded band inversion. f, Spectrum of the same model solved on a nanowire with hexagonal cross-section and periodic boundary conditions in the trigonal z direction ([111] direction). Here, k_z denotes the momentum along this direction. Only a portion of the spectrum at small momentum deviations from the T point k_z = π is shown. Six Kramers pairs of hinge modes traverse the surface and bulk gap. See Supplementary Fig. 2c for a zoomed-out version showing the spectrum for all momenta. g, Localization of these topologically protected hinge modes in the x–y plane.

Thus far, our considerations apply to all crystals with TRS, $\hat C_3$ and $\hat I$. We now evaluate the above topological index for elementary bismuth, crystallizing in space group $R\bar 3m$, number 166, which possesses these symmetries (see Fig. 1b). Even though bismuth is not an insulator, there exists a direct bandgap separating valence bands from conduction bands (see Fig. 1e). This allows us to evaluate the indices v^(π) and v^(±π/3) for the valence bands. We do so with the group characters obtained from first-principles calculations (see Methods). The result is v^(π) = v^(±π/3) = −1, which derives from $\nu _T^{({\mathrm{\uppi}} )} = \nu _T^{( \pm {\mathrm{\uppi}} /3)} = - 1$, that is, there is a $\hat C_3$-graded double band inversion at the T point. Hence, bismuth is a HOTI according to the topological index defined above (if we neglect the fact that it has a small electron and hole pocket).

As a second approach, we employ the formalism of elementary band representations^{45,46,47,48,49,50} to demonstrate the nontrivial topology. Since there is always an energy separation between valence and conduction bands, we restrict our consideration to the three doubly-degenerate valence bands shown in red in Fig. 1e. In particular, we checked explicitly that the set of all bands at lower energy than these is topologically trivial. At time-reversal symmetric momenta the eigenvalues of all symmetry operators have been computed (see Methods). Referring to the character tables in the Bilbao Crystallographic Server⁴⁷, we assign to all the bands their corresponding irreducible representations. The results of the eigenvalue calculations are listed in Supplementary Information section C. They show that the valence bands cannot be decomposed into any linear combination of physical elementary band representations, which are elementary band representations that respect TRS. It is the main result of ref. ⁴⁵, that if such a decomposition is not possible, the electronic band structure of bismuth has to be topological and without a description in terms of exponentially localized Wannier states, in contraposition to the conclusion drawn from Fu–Kane’s parity criterion⁵. To understand which symmetry protects this topological phase, we repeated the symmetry eigenvalue calculation with an artificially lowered symmetry. The representative elements of point group $\bar 3m$ are $\hat C_3$ around the z axis (denoted 3 in the space group names), $\hat I$ (denoted by overbar), two-fold rotational symmetry about the y axis (denoted 2), and mirror symmetry with respect to the x–z plane (denoted m). After lowering the space group $R\bar 3m$ (166) to R3m (160) or R32 (155), a similar elementary band representation analysis within the symmetry-reduced space groups shows that the valence bands can be decomposed into physical elementary band representations in this case, indicating that they are topologically trivial. Therefore, neither two-fold rotation nor mirror symmetry protects the nontrivial topology of bismuth. In contrast, as long as $\hat I$ is preserved, lowering it to space group $R\bar 3$ (148), the valence bands are still topological in the sense that they cannot be decomposed into physical elementary band representations in space group 148. We conclude that the nontrivial topology is protected by $\hat I$ (in combination with the three-fold rotation). Notice that the rhombohedral lattice always respects the three-fold rotational symmetry. Since we learned from topological quantum chemistry that the bulk bands have no Wannier description, we expect the presence of spectral flow in Bi, and hence protected gapless modes on its boundaries. Since we know the surfaces of bismuth to be non-topological, these gapless boundaries must be hinges. This is compatible with previous works showing that Bi (111) bilayers (possibly on a substrate) host one-dimensional edge channels.^42,43

When we change the parameters of the tight binding-model of bismuth⁵⁷ slightly, it undergoes a transition from a second-order to a first-order topological insulator⁵⁸. However, we confirmed the higher-order character of bismuth that is suggested by the original tight-binding model parameters⁵⁷ independently by performing first-principles calculations, as well as an analysis in the framework of topological quantum chemistry. In particular, we took into account all occupied bands of bismuth up to its momentum-dependent energy gap. This is important since it has been shown that bands far away from this gap still contribute significantly to measurable effects, such as the unusually large g-factor of holes⁵⁹.

Bulk–boundary correspondence

We present a direct calculation which allows us to conclude that a TRS system with v^(π) = v^(±π/3) = −1 has to have hinge modes for terminations of the crystal that globally respect inversion symmetry or further symmetries. We consider a crystal of hexagonal shape (see Fig. 1c) that preserves $\hat C_3$ rotational and inversion symmetry. The steps outlined here in words are explicitly demonstrated using a Dirac model in Supplementary Information section A. We think of the insulator with v^(π) = v^(±π/3) = −1 as a superposition of two topological insulators, one in each of the independent $\hat C_3$ subspaces. Consider adiabatically turning off any coupling between these two subspaces, while preserving the bulk gap. The resulting system has two Dirac cones (a Dirac theory represented by 4 × 4 matrices) on all surfaces of the crystal. Next, we seek to gap these surface Dirac cones by weakly coupling the two $\hat C_3$ subspaces. We want to do so while preserving TRS, as well as the symmetries $\hat C_3$ and $\hat I$ of the crystal. Of these, TRS is the only constraint that acts locally on a given surface. From the representation theory of the two-dimensional Dirac equation, we find that for a TRS that squares to −1, as required for spinful electrons, there exists a unique mass term m that gaps the two Dirac cones in a time-reversal symmetric way. It remains to study how this mass term transforms under $\hat C_3$ and $\hat I$ to determine its relative sign between different surfaces of the crystal. Relative to the kinetic part of the surface Dirac theory, m → −m under inversion and m → +m under $\hat C_3$ (see Supplementary Information section A for details). As a result, the sign of the mass term alternates between adjacent lateral surfaces of the hexagonal crystal (see Fig. 1c). Each change of sign in the mass term is a domain wall in the Dirac theory and binds a Kramers pair of modes propagating along it. These are the one-dimensional hinge modes of the HOTI. The sign of the mass term on the top and bottom surface is not universally determined so that both patterns of hinge modes shown in Fig. 1c are compatible with the bulk topology of v^(π) = v^(±π/3) = −1 (in a real system, the particular electronic structure determines which pattern has lower energy). Apart from this ambiguity, the argument presented here rests solely on the nontrivial bulk topology and is independent of the exact form of the surface electronic structure, as long as the surface is gapped while preserving the respective symmetries. This constitutes the generalized topological bulk–boundary correspondence characteristic of a HOTI, where the existence of one-dimensional hinge modes directly follows from the three-dimensional bulk topology. The HOTI’s bulk–boundary correspondence requires that these hinge modes are locally stable under time-reversal symmetric perturbations that preserve the bulk and surface gaps. From this requirement, we can understand the ${\Bbb Z}_2$ topological character of the phase: the minimal TRS surface manipulation is the addition of a two-dimensional topological insulator to one surface of the hexagonal nanowire. This would permit hybridizing and gapping out of the pair of hinge modes adjacent to the surface. However, to comply with $\hat I$ and $\hat C_3$, the same two-dimensional topological insulator has to be added to every surface, thus leaving the Kramers pairs of modes intact at each hinge. We conclude that a single Kramers pair of modes at each hinge is stable under all symmetry-preserving surface perturbations. In fact, such a Kramers pair is locally stable under small perturbations even when the spatial symmetries are broken, for example, by introducing disorder into the sample, as long a TRS is preserved. The only way to remove it is to annihilate it with another Kramers pair coming from another hinge, which cannot be achieved with just a small perturbation. The higher-order hinge modes of a three-dimensional HOTI are therefore just as stable as the edge modes of a first-order TRS topological insulator in two dimensions. We further exemplify these results with a tight-binding model, defined in Supplementary Information section B, whose hinge states are shown in Fig. 1d,f,g. We note that our tight-binding model is topologically equivalent to a realistic model⁵⁷ of bismuth, but it is easier to interpret in the sense that it does not have metallic bulk and surface states that would obscure the hinge modes in the electronic structure plots we present here. It also has fewer orbitals per unit cell, which makes three-dimensional simulations of large systems feasible.

We now turn to experimental data that support our higher-order bulk–boundary correspondence in bismuth. Even though bismuth is metallic in the bulk and on the surface, only its topological hinge states are protected against scattering by weak disorder as compared to trivial surface states, for example. We expect hinge states between (i) the top surface, which is denoted (111) in the primitive unit vectors, and three of the six lateral surfaces and (ii) between adjacent lateral surfaces. The geometry of the samples was more amenable to the study of the hinge states of type (i), as we outline below.

STM experiment

With an STM, we studied the electronic structure of step edges on the (111) surface of bismuth. Owing to the buckled honeycomb structure of the bismuth bilayer along the [111] trigonal direction, STM topographic images of the (111) plane of bismuth show bilayer steps with two different types of bisectrix edges: type A and type B (marked as red and blue lines in Fig. 2a). We highlight two structures of triangular and nearly hexagonal shape (Fig. 2a,c). In particular the step edge in Fig. 2c can be seen as (the negative of) a one bilayer tall version of the crystal shapes shown in Fig. 1c. We thus expect hinge states at either the type A or the type B edges owing to the higher-order topology. (All A type and all B type edges are mutually equivalent owing to the $\hat C_3$ rotational symmetry of the bismuth (111) surface.) Indeed, we observe strongly localized edge states only at type A edges in Fig. 2b,d, which display the differential conductance map overlaid on top of the topographic data to illuminate the edge states at the van Hove singularity energy of the bismuth edge states. A previous experimental study³⁸ showed a one-dimensional van Hove singularity of the edge states (E = 183 meV) and quasi-particle interference of the spin-orbit locked edge states. The same study demonstrated the absence of k to −k scattering for these states. These experimental observations and model calculations strongly suggest that the edge states are living in the momentum-dependent energy gap of the bismuth (111) surface states³⁸. Every other edge of a hexagonal pit exhibits localized edge states and these edge states are discontinued at the corner where type A and type B edge meet (Fig. 2c,d). This feature remarkably reproduces the hinge modes calculated for the hexagonal nanowire, as shown in Fig. 1d.

Fig. 2: Experimental observation of the alternating edge states on a bismuth (111) surface perpendicular to its trigonal axis.

a, Three-dimensional rendered topographic image of the bismuth (111) surface. The red (type A) and blue (type B) lines then indicate the types of edge, which are along bisectrix axes. The edges of type B in this particular pit geometry are much shorter than edges of type A, while still large enough to be experimentally accessible. b, Differential conductance map at the van Hove singularity energy (V = 183 meV) of the one-dimensional edge states. In contrast to the type B edges, all the type A edges exhibit localized high conductance. c, Topographic image of a hexagonal pit on a bismuth (111) surface. The hinge modes are schematically shown as purple lines. Blue and red arrows indicate the flow of the spin–momentum locked hinge modes. d, Differential conductance map simultaneously acquired with the topographic data from c, showing high conductance at every other edge of the hexagonal pit.

Transport experiment

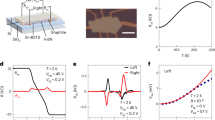
We exploited proximity-induced superconductivity to reveal ballistic hinge states along monocrystalline bismuth nanowires^39,40. When these (non-superconducting) nanowires are connected to superconducting contacts (implementing a superconductor/bismuth nanowire/superconductor or S/Bi/S Josephson junction), a supercurrent runs through them at low temperature. Our experiments unambiguously demonstrate that the supercurrent flows via extremely few narrow one-dimensional channels, rather than via the entire surface or bulk of the nanowire. The experimental indications are as follows. (i) Periodic oscillations of the critical current through the nanowires caused by a magnetic field, with a period corresponding to one magnetic flux quantum through the wire section perpendicular to the field^39,40. Such oscillations indicate interference between two supercurrent-carrying paths located at the nanowire edges⁶⁰ (see also the Supplementary Information), since a uniform current density in such a long narrow wire would produce instead a monotonously decaying critical current. (ii) The supercurrent flowing through the nanowire persists to extremely high magnetic fields, up to several Tesla in some samples. Since the orbital dephasing due to a magnetic flux through the supercurrent-carrying channel area destroys the induced supercurrent, this indicates that the channels are extremely narrow spatially. (iii) Finally, we have recently provided a direct signature of ballistic transport along those one-dimensional channels, by measuring the supercurrent-versus-phase relation (also called current–phase relation) of the S/Bi/S junction. This was done by inserting the bismuth nanowires into an asymmetric superconducting quantum interference device (SQUID) configuration^40,61. Whereas tunnelling or diffusive transport give rise to the usual nearly sinusoidal current–phase relation of superconductor/normal metal/superconductor Josephson junctions, the sharp sawtooth-shaped current–phase relation that we found instead demonstrates that transport occurs ballistically along the wire. The scattering probability p was estimated to be 0.1 along the 1 μm long bismuth wire from the harmonics content of this current–phase relation (where the nth harmonic decays like (1−p)²ⁿ/n). This leads to a lower bound of the elastic mean free path l_e along these edges equal to 10, much longer than the value l_e = 0.1 determined for the surface states. This surprising result is explained by the dominant contribution of the topologically protected hinge states to the supercurrent. Indeed, the supercurrent carried by a diffusive channel is (L/l_e)² ≈ 100 times smaller than the supercurrent carried by a ballistic channel (L is the wire length). The position of the edge states can be deduced from the periodicity of the SQUID oscillations, which is inversely proportional to the area enclosing the flux. In a sample of parallelogrammatic cross-section whose geometry and orientation was precisely determined, we detected a beating of two paths enclosing different fluxes $\varPhi$ and $\varPhi ^\prime$ (see Fig. 3a)⁴⁰. This demonstrated that the edge states are located along the two acute edges of the (111) facets. Those edges coincide with the expected hinge states perpendicular to the trigonal [111] axis (see Fig. 3b). The contribution of each path was extracted and is shown in Fig. 3d,e. The supercurrents carried by the two hinges differ by a factor of four. This can be explained by a difference in the quality of the contact to these hinge states: the top hinges of the wire have been more severely etched than the bottom ones during the deposition of the superconducting electrodes (see Fig. 3a). This strong etching reduces the coupling of edge states to the superconducting contacts and the supercurrent is decreased even though the ballistic nature is unaffected.

Fig. 3: Evidence for hinge states from Josephson-interference experiments.

a, Single-crystal bismuth nanowire (coloured brown) connected to superconducting electrodes (coloured blue). The wire has a parallelogrammatic cross-section. Its orientation along one of the bisectrix axes of bismuth was determined by electron diffraction, showing evidence of (111) facets parallel to the substrate. The 1.4 μm long, rightmost section of the wire, in parallel with a superconducting weak link, forms an asymmetric SQUID. b, Schematic representation of the investigated bismuth nanowire of parallelogrammatic cross-section described above, indicating (red lines) the position of the experimentally identified topological hinge states in relation to the hinge states determined theoretically in a bismuth sample of hexagonal symmetry oriented along the trigonal [111] axis. c, The magnetic field B dependence of the critical current I_c shown is modulated by the current–phase relation of the bismuth Josephson junction (whose critical current is much lower than the superconducting weak link). d,e, The current–phase relation in c can be decomposed into the sum of two sawtooth waves of different periods, corresponding respectively to the internal and external area of the SQUID $\varPhi$ and $\varPhi ^\prime$ shown in a.

Comparing Figs. 3d and 1c, we note that one of the two hinges on top of the nanowire must be of A type and the other one of B type (the same is true for the bottom two hinges). Our observation of a ballistic channel at one of these hinges at the top, and one at the bottom of the nanowire, is thus in line with the theoretical expectation from the higher-order topology of bismuth.

Summary

The bismuth–antimony alloy, Bi_1−xSb_x, was the first material realization of a three-dimensional topological insulator^3,5. The composition x was used to interpolate between bismuth, without band inversion, and the band-inverted antimony. In this work, we have demonstrated theoretically that the allegedly trivial end of this interpolation, bismuth, has in fact a three-dimensional topological band structure as well. It is a HOTI with helical hinge states. We have presented two complementary pieces of experimental evidence supporting this result, using STM and Josephson-interferometry measurements. The type of hinge states discussed here may be used for lossless electronic transport owing to their local protection from backscattering by TRS disorder. Further applications include spintronics, owing to their spin–momentum locking, and—when proximitized with superconductivity—topological quantum computation. For the latter, a nanowire with hexagonal cross-section may provide a particularly convenient way of building a hexon: a group of six Majorana states, one at each hinge. Hexons have been proposed as building blocks for a measurement-only quantum computer⁶².

Methods

First-principles calculations

We employed density functional theory as implemented in the Vienna Ab Initio Simulation Package (VASP)^64,65,66,67. The exchange correlation term is described according to the Perdew–Burke–Ernzerhof prescription, together with projected augmented-wave pseudopotentials^68,69 and the spin–orbit interaction included. For the self-consistent calculations we used a 12 × 12 × 12 k-point mesh for the bulk band-structure calculations. The eigenvalues of the symmetry transformations were deduced from the matrix representations of the respective symmetry operation calculated using the Bloch eigenstates from VASP.

STM experiment

Bismuth crystals were cleaved at room temperature under ultrahigh vacuum conditions and the cleaved samples were cooled down to 4 K, at which temperature STM and STS measurements were carried out. The cleaved bismuth crystal exhibits a (111) plane of the bismuth rhombohedral structure (which is the (001) plane of the bismuth hexagonal structure). For STM measurements, a mechanically sharpened platinum–iridium tip was used, and electronic properties of the probe tip were characterized before the experiments on bismuth by checking a reference sample. Differential conductance maps (Fig. 2b,d) were taken simultaneously with topographic data at the van Hove singularity energy (V = 183 meV) of the bismuth edge states using a lock-in amplifier with an oscillation of 3 meV and current I = 3.5 nA. The data shown in this work is reproduced on many step edges of Bi (111) with atomically different tips. All of the islands on the Bi (111) surface show the expected step height of 4 Å for bismuth bilayers and all of the extended edges are identified as zigzag structures of either A type or B type. A type and B type edges are equivalent in the hexagonal nanowire geometry as described in the main text (Fig. 1c); however, the existence of the Bi (111) surface under the bismuth bilayer breaks the inversion symmetry, and A as well as B type edges can be identified in STM measurements. Only A type edges show the spectroscopic feature of a sharp peak at 183 meV, which is the van Hove singularity energy of the one-dimensional edge state. Quasi-particle interference measurements reveal that this edge state is continuously dispersing down to the Fermi level and starts to merge with the surface states at the momentum where the surface gap closes³⁸. This spectroscopic feature of geometric confinement only at A type edges resembles the topological hinge modes expected for the hexagonal nanowire, as discussed in the main text.

Transport experiment

The nanowires grew during slow sputtering deposition of high-purity bismuth on a slightly heated silicon substrate. High-resolution transmission electron microscopy indicates high-quality single crystals of hexagonal or rhombohedral cross-sections, with clear facets. The facet widths are typically 50 nm to 300 nm wide. Resistance measurements show that transport in the normal state (when contacts to the nanowires are not superconducting) occurs predominantly due to surface states, with an elastic mean free path of the order of 100 nm.

Data availability

The data that support the plots in Figs. 1, 2 and 3 within this paper and other findings of this study are available from the corresponding author upon reasonable request. The information on elementary band representations is available on the Bilbao crystallographic server⁶³.